Checking Symmetries

In the following Mathlet, use the color palette to design the square on the left. Use the drop-box to select a transformation, and notice the result on the right-hand side.

Important Concept: A transformation is a symmetry for a square design if after you apply the transformation to the design, the design appears unchanged.

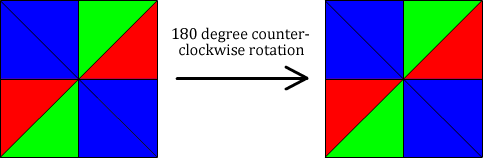
For example, consider the two transformations shown below.

Since the square design doesn't change when it is rotated 180 degrees counter-clockwise, we say that "180 degree counter-clockwise rotation" is a symmetry for this design.

Since the square design changes when it is flipped over a vertical line, we say that "vertical line flip" is not a symmetry for this design.

Each of the eight basic operations is potentially a symmetry for each square design, but depending on how you design your square, the actual symmetries will vary. Explore with this Mathlet and see what kinds of squares you can design.

Challenges:

1. Can you design a square that has only one symmetry? What does this one symmetry have to be?
2. Can you design a square that has only two symmetries? Can you have any combination of two symmetries?
3. Can you design a square that has only three symmetries?
4. Can you design a square that has only rotational symmetries?
5. Can you design a square that has only flip symmetries?