| Angle | Degrees | Radians | cosine | sine | tangent |
|---|---|---|---|---|---|
 | 90° | 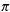 /2 /2 | 0 | 1 | infinity |
 | 60° | 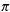 /3 /3 | 1/2 | 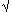 3 / 2 3 / 2 | 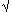 3 3 |
 | 45° | 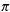 /4 /4 | 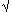 2 / 2 2 / 2 | 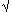 2 / 2 2 / 2 | 1 |
 | 30° | 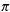 /6 /6 | 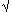 3 / 2 3 / 2 | 1/2 | 1/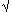 3 3 |
| 0° | 0 | 1 | 0 | 0 |




But ED = tan A, AE = 1, CB = sin A, and AC = cos AB. Therefore we have derived the fundamental identity
| tan A = | sin A cos A |
| tan = | opp adj |
The point B is where the line cuts the y-axis. We can let the coordinates of B be (0,b) so that b, called the y-intercept, indicates how far above the x-axis B lies. (This notation conflicts with labelling the sides of a triangle a, b, and c, so let's not label the sides right now.)
You can see that the point 1 unit to the right of the origin is labelled 1, and its coordinates, of course, are (1,0). Let C be the point where that verical line cuts the horizontal line through B. Then C has coordinates (1,b).
The point A is where the vertical line above 1 cuts the original line. Let m denote the distance that A is above C. Then A has coordinates (1,b+m). This value m is called the slope of the line. If you move right one unit anywhere along the line, then you'll move up m units.
Now consider the angle CBA. Let's call it the angle of slope. It's tangent is CA/BC = m/1 = m. Therefore, the slope is the tangent of the angle of slope.
The term "angle of elevation" refers to the angle above the horizontal from the viewer. If you're at point A, and AH is a horizontal line, then the angle of elevation to a point B above the horizon is the angle BAH. Likewise, the "angle of depression" to a point C below the horizon is the angle CAH.
Tangents are frequently used to solve problems involving angles of elevation and depression.
Note that the tangent of a right angle is listed as infinity. That's because as the angle grows toward 90°, it's tangent grows without bound. It may be better to say that the tangent of 90° is undefined since, using the circle definition, the ray out from the origin at 90° never meets the tangent line.
| Angle | Degrees | Radians | cosine | sine | tangent |
|---|---|---|---|---|---|
 | 90° | 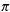 /2 /2 | 0 | 1 | infinity |
 | 60° | 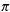 /3 /3 | 1/2 | 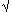 3 / 2 3 / 2 | 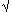 3 3 |
 | 45° | 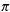 /4 /4 | 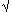 2 / 2 2 / 2 | 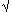 2 / 2 2 / 2 | 1 |
 | 30° | 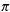 /6 /6 | 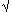 3 / 2 3 / 2 | 1/2 | 1/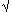 3 3 |
| 0° | 0 | 1 | 0 | 0 |
29. In a right triangle a = 30 yards and tan A = 2. Find b and c.
49. cos t = 2 tan t. Find the value of sin t.
Note: In the following problems distance means horizontal distance, unless otherwise stated; the height of an object means its height above the horizontal plane through the point of observation. The height of the observer's eye is not to be taken into account unless specially mentioned. In problems involving the shadow of an object the shadow is supposed to fall on the horizontal plane through the base of the object, unless otherwise stated.
151. The angle of elevation of a tree 250 feet distant is 16° 13'. Find the height.
152. Find the height of a steeple distant 321 feet, angle of elevation 35 ° 16'.
153. From a ship, the angle of elevation of the top of a lighthouse 200 feet above the water is 2° 20'. Find the distance.
154. From the top of a lighthouse 165 feet above the water the angle of depression of a ship is 3° 50'. Find the distance.
159. Find the height of a tower, distant 186 feet, angle of elevation 40° 44'.
160. On one side of a stream a pole 50 feet high has from an opposite point an angle of elevation of 5° 33'. Find the breadth of the stream.
164. From one hill the top of another 128 feet higher has an angle of elevation of 2° 40'. Find the distance.
165. From one hill to the top of another distant 6290 feet has an angle of elevation of 4° 9'. Find how much the height of the second hill exceeds that of the first.
189. The gable end of a roof measures 40 feet across at the base and 26 feet from the base to the ridge. At what angle do the rafters slope?
29. Since you know a and tan A, you can find b. You can then determine c by the Pythagorean theorem, or by using sines, or by using cosines.
49. You'll need two identities. First, tan t = sin t/cos t. Second, the Pythagorean identity, sin2 t + cos2 t = 1. Then you'll have to solve a quadratic equation.
151. Remember that the tangent of an angle in a right triangle is the opposite side divided by the adjacent side. You know the adjacent side (the distance to the tree), and you know the angle (the angle of elevation), so you can use tangents to find the height of the tree.
152. You know the angle (again, the angle of elevation) and the adjacent side (the distance to the steeple), so use tangents to find the opposite side.
153. Using the angle and the opposite side, use tangent to find the adjacent side.
154. Same hint as in 153.
159. Same hint as in 152.
160. Same hint as in 153.
164. Same hint as in 153.
165. Same hint as in 152.
189. The gable end of a roof is an isosceles triangle. If you drop a perpendicular line from the ridge, you get two congruent right triangles. You know the two legs of the triangles, so you can determine the angle of slope of the rafters using arctangent.
29. b = a/tan A = 30/2 = 15 yards. c = 33.5 yards.
49. Since cos t = 2 tan t, therefore cos t = 2 sin t/cos t, so cos2 t = 2 sin t, and, by the Pythagorean identity, you get 1 – sin2 t = 2 sin t.
That gives you a quadratic equation
sin2 t + 2 sin t – 1 = 0.
The solutions are sin t = –1 ± 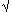 2. Of these two solutions the only feasible one is
sin t =
2. Of these two solutions the only feasible one is
sin t = 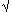 2 – 1.
2 – 1.
151. Height = 250 tan 16°13' = 72.7' = 72'9".
152. Height = 321 tan 35°16' = 227 feet.
153. Distance = 200/tan 2°20' = 4908 feet, nearly a mile.
154. Distance = 165/tan 3°50' = 2462 feet, nearly half a mile.
159. Height = 186 tan 40°44' = 160 feet.
160. Distance = 50/tan 5°33' = 515 feet.
164. Distance = 128/tan 2°40', about 2750 feet, a little more than half a mile.
165. Height = 6290 tan 4°9' = 456.4 feet.
189. tan A = 26/20, so A = 52°.

Table of contents. | Back to cosines. | On to right triangles.

David E. Joyce
Department of Mathematics and Computer Science
Clark University
Worcester, MA 01610
Email: djoyce@clarku.edu
Dave's Short Trig Course is located at http://aleph0.clarku.edu/~djoyce/java/trig