There is one more kind of limit that I will cover now briefly. The limit concept is the same; it's just another way that we can approach the limit. Consider the function:
x2 - 1
f(x) = eq. 2.3-1
x2 + 1
This function is graphed here in figure 2.3-1.
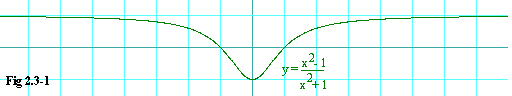Notice that as x gets bigger (that is as you go to the
right on the graph), f(x) appears to get closer and closer
to 1. If you plug in a few values, you see the same trend:
f(1) = 0 tab. 2.3-1 f(2) = 0.6 f(5) = 0.9231 f(10) = 0.9802 f(100) = 0.9998 f(1000) = 0.999998We have a feeling that this function can get as close to 1 as you'd like provided you choose x big enough (or small enough for that matter. The function seems to have the same behavior as x grows more and more negative). That statement sounds so similar to the contracts that we have been talking about for limits that we ought to be able to apply the same line of thought. And indeed we can.
So how close does f(x) get to 1? We find that by taking the absolute value of the difference. After you put both terms over a common denominator, you get:
2
|f(x) - 1| = eq. 2.3-2
x2 + 1
The denominator clearly gets big without limit as x gets big without
limit. And the
numerator is constant. So we can expect the difference to get closer
and closer to zero as x gets big. In fact, if you wanted
the difference to be at least as small as some
æ 2 ö
x ³ sqrtç - - 1 ÷ eq. 2.3-3
è e ø
and you'll be guaranteed it." (noting that e must be less than
2. If it isn't, then any x can get you within
e). Starting to sound familiar? And do please
try to reproduce for yourself the algebra that I used to get 2.3-3 from
2.3-2.
This is a situation where f(x) approaches a limit as x grows without limit, or, in the popular nomenclature, as x goes to ¥ (which is the symbol for infinity). So we say:
lim f(x) = L eq. 2.3-4 xto mean that for any> ¥
|f(x) - L| £ e eq. 2.3-4whenever
Here is a useful trick for taking a limit as x goes to infinity. Supose you had, for example
x (1 - x)
lim eq. 2.3-5
x > ¥ (2 + x)(3 + x)
If you substitute
1
x = eq. 2.3-6
u
throughout and then take the limit as u goes to zero, you
will get the same thing. Why? Because u does go to
zero as x goes to infinity. In this example you get:
1 æ 1 ö
ç 1 - ÷
u è u ø
lim eq. 2.3-7
u > 0 æ 1 ö æ 1 ö
ç 2 + ÷ ç 3 + ÷
è u ø è u ø
At first, this looks pretty nasty, but if you multiply numerator and
denominator by u2, it gets much better. Notice that
each of the factors in the numerator get multiplies by a factor of u,
and likewise each of the factors in the denominator.
(1) (u - 1)
lim eq. 2.3-8
u > 0 (2u + 1)(3u + 1)
Since this thing is already factored for you, you can apply the product
rule for limits (remember, the limit of a product is the product of
the limits). So take the limits:
1
lim eq. 2.3-9a
u > 0 2u + 1
and
u - 1
lim eq. 2.3-9b
u > 0 3u + 1
These two are each pretty easy. If you put zero in for u, neither
of them gives you any trouble -- no zeros in the denominator that is.
I get 1 for the limit
of the first one and -1 for the limit of the second. The
product is -1, so that is also the limit of eq. 2.3-7 as
u goes to zero as well as the limit of eq. 2.3-5 as x
goes to infinity.
This method always works for finding limits as something goes to infinity. Substitute 1/u for that something everywhere it occurs, then take the limit as u goes to zero using whatever methods are in your arsenal for taking such limits. Often you will have to simplify first by multiplying numerator and denominator by some power of u in order to clear the little fractions. But you learned how to do that in algebra.
You could even do a delta-epsilon proof of the limit as u
goes to zero. Since x goes to infinity as u goes to zero,
you can argue that showing that finding a d close enough to
zero to meet the e contract for the u-version
is the the problem
same as finding an x large enough to meet the same e
contract for the x-version of the problem. In fact, whenever
1) See if you can come up with the definition of what it means for a function, f(x), to have a limit as x goes to -¥ (that is, as x gets negative without limit). Apply that definition to the function in 2.3-1, and show that it, indeed, approaches a limit of 1 as x goes to -¥.
2) Find and prove the limit of
3x + 3
f(x) =
x - 1
as x goes to ¥
email me at hahn@netsrq.com